MATHEMATICS; STANDARD NOTATION ( NUMBERS)

STANDARD NUMBERS SIMPLIFIED;

Scientific Notation (also called Standard Form in Britain) is a special way of writing numbers:

Like this:
Or this:

It makes it easy to use big and small values.

OK, How Does it Work?

Example: 700

Why is 700 written as 7 × 10² in Scientific Notation ?

700 = 7 × 100

and 100 = 10² (see powers of 10)

so 700 = 7 × 10²

Both 700 and 7 × 10² have the same value, just shown in different ways.

Example: 4,900,000,000

    1,000,000,000 = 10⁹ ,
so 4,900,000,000 = 4.9 × 10⁹ in Scientific Notation

The number is written in two parts:

• Just the digits, with the decimal point placed after the first digit, followed by
• × 10 to a power that puts the decimal point where it should be
  (i.e. it shows how many places to move the decimal point).

In this example, 5326.6 is written as 5.3266 × 10³,
because 5326.6 = 5.3266 × 1000 = 5.3266 × 10³

Try It Yourself

Enter a number and see it in Scientific Notation:

Enter Number:

Scientific Notation:

3.1416 × 10⁰

© 2015 MathsIsFun.com v 0.85

Now try to use Scientific Notation yourself:

Scientific Notation:

× 10

Number:

314.16

© 2015 MathsIsFun.com v 0.85

Other Ways of Writing It

3.1 × 10^8

We can use the ^ symbol (above the 6 on a keyboard), as it is easy to type.

Example: 3 × 10^4 is the same as 3 × 10⁴

• 3 × 10^4 = 3 × 10 × 10 × 10 × 10 = 30,000

Calculators often use "E" or "e" like this:

Example: 6E+5 is the same as 6 × 10⁵

• 6E+5 = 6 × 10 × 10 × 10 × 10 × 10 = 600,000

Example: 3.12E4 is the same as 3.12 × 10⁴

• 3.12E4 = 3.12 × 10 × 10 × 10 × 10 = 31,200

How to Do it

To figure out the power of 10, think "how many places do I move the decimal point?"

	When the number is 10 or greater, the decimal point has to move to the left, and the power of 10 is positive.
	When the number is smaller than 1, the decimal point has to move to the right, so the power of 10 is negative.

Example: 0.0055 is written 5.5 × 10^-3

Because 0.0055 = 5.5 × 0.001 = 5.5 × 10^-3

Example: 3.2 is written 3.2 × 10⁰

We didn't have to move the decimal point at all, so the power is 10⁰

But it is now in Scientific Notation

Check!

After putting the number in Scientific Notation, just check that:

• The "digits" part is between 1 and 10 (it can be 1, but never 10)
• The "power" part shows exactly how many places to move the decimal point

Why Use It?

Because it makes it easier when dealing with very big or very small numbers, which are common in Scientific and Engineering work.

Example: it is easier to write (and read) 1.3 × 10^-9 than 0.0000000013

It can also make calculations easier, as in this example:

Example: a tiny space inside a computer chip has been measured to be 0.00000256m wide, 0.00000014m long and 0.000275m high.

What is its volume?

Let's first convert the three lengths into scientific notation:

• width: 0.000 002 56m = 2.56×10^-6
• length: 0.000 000 14m = 1.4×10^-7
• height: 0.000 275m = 2.75×10^-4

Then multiply the digits together (ignoring the ×10s):

2.56 × 1.4 × 2.75 = 9.856

Last, multiply the ×10s:

10^-6 × 10^-7 × 10^-4 = 10^-17 (easier than it looks, just add −6, −4 and −7 together)

The result is 9.856×10^-17 m³

It is used a lot in Science:

Example: Suns, Moons and Planets

The Sun has a Mass of 1.988 × 10³⁰ kg.

Easier than writing 1,988,000,000,000,000,000,000,000,000,000 kg
(and that number gives a false sense of many digits of accuracy.)

Play With It! Use Scientific Notation 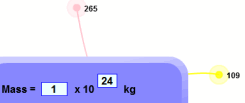in Gravity Freeplay

 

It can also save space! Here is what happens when you double on each square of a chess board:

Values are rounded off, so 53,6870,912 is shown as just 5×10⁸

That last value, shown as 9×10¹⁸ is actually 9,223,372,036,854,775,808

Engineering Notation

Engineering Notation is like Scientific Notation, except that we only use powers of ten that are multiples of 3 (such as 10³, 10^-3, 10¹² etc).

Examples:

• 2,700 is written 2.7 × 10³
• 27,000 is written 27 × 10³
• 270,000 is written 270 × 10³
• 2,700,000 is written 2.7 × 10⁶

Example: 0.00012 is written 120 × 10^-6

Notice that the "digits" part can now be between 1 and 1,000 (it can be 1, but never 1,000).

The advantage is that we can replace the ×10s with Metric Numbers. So we can use standard words (such as thousand or million), prefixes (such as kilo, mega) or the symbol (k, M, etc)

Example: 19,300 metres is written 19.3 × 10³ m, or 19.3 km

Example: 0.00012 seconds is written 120 × 10^-6 s, 

https://www.mathsisfun.com/numbers/scientific-notation.html